Miscellaneous

Elementary
GCD
LCM
Definitions

Percents

Ratios

Square Roots

Reverses

Junior High
Cube Roots

Mult By 9 (#1)
Mult By 9 (#2)

Mult By 8

Polynomials

Positive Integral Divisors

Sum Of Positive Integral Divisors

Relatively Prime 

Triangular Numbers

Remainders

Divide By 40

Divide By 25

Divide By 9

High School

Pentagonal Numbers

Hexagonal Numbers

Heptagonal Numbers

Octagonal Numbers

Manipulating Polygonal Numbers

Dividing Factorials #1

Dividing Factorials #2

Solving Triangular Numbers

(*View/Download .pdf file*)

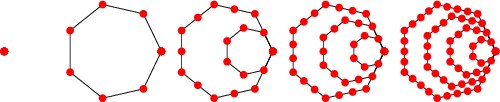
Heptagonal Numbers:

A.  Heptagonal Numbers are numbers that create a heptagon.  In other words: 1, 7, 18, 34, etc.

B.  The n^th heptagonal number can be found by the formula:

n(5n - 3)

2

C.  In number sense, the question will only ask for the n^th heptagonal number.

Ex [1]  The 6^th heptagonal number is __________.

a.  Using the formula we get: ^(6)(27)/₂ or 3 x 27 = 81.

b.  The answer is 81.

Ex [2]  The 10^th heptagonal number is __________.

a.  Using the formula we get: ^(10)(47)/₂ or 5 x 47 = 235.

b.  The answer is 235.

D.  Here are some ways of manipulating heptagonal numbers:

1.  The difference of successive heptagonal numbers is:

5n - 4, where n is the largest

2.  Adding successive heptagonal numbers gives:

3n² - 4n + 2, where n is the largest

NOTE:  You might see #1 on a test, but I doubt you will ever see #2 on a test.

Back to top